GREPhysics.NET: GR9677 #74

GR9677 #74

Problem

GREPhysics.NET Official Solution

This problem is still being typed.

Thermodynamics $\Rightarrow$ }Entropy

Recall the definition of entropy to be $dS = dQ/T$ . The heat is defined here as $dQ = m c dT$ , and thus $S = \int mcdT/T$ .

One is given two bodies of the same mass. One mass is at $T_1=500$ and the other is at $T_2=100$ before they're placed next to each other. When they're put next to each other, one has the net heat transferred being 0, thus $Q_1 = -Q_2 \Rightarrow T_f = (T_1+T_2)/2=300$ .

The entropy is thus $S = \int^{T_f}_{T_1} mcdT/T + \int^{T_f}_{T_2} mcdT/T = mc \left ( \ln(3/5) + \ln(3) \right) = 2mc \ln 3 - mc \ln 5 = mc(\ln 9 - \ln 5) = mc \ln(9/5)$ , as in choice (B).

Alternate Solutions

asdfman
2009-11-05 00:11:38

Used POE.

Expect there to be a change in entropy - E is out.
Know the typical integral to find S will involve a $ln$ - A is out.
Expect the entropy to increase for the system - D is out.
Based on the numbers, expect there to be a ratio as an argument of the $ln$ - C is tentatively out.

I'd come back to it if there was time and run the math to see if B is actually correct. If not this logic narrows it down to 2 choices.

Reply to this comment

Comments

q
2019-10-15 15:39:09

Cependant, Hernandez ne re?oit pas suffisamment l\'occasion de montrer ses compétences comme il joue derrière Ronaldo, Gareth Bale, Karim Benzema et James Rodriguez.\r\nq http://www.alaubergededaon.com/fr2.asp

Reply to this comment

q
2019-10-09 14:08:02

Sevilla: 12-2-4, 39 pointsFollow Damon Salvadore sur TwitterFOR PLUS NOUVELLES ET MISES à JOUR SPORTS, cliquez sur ce lien pour visiter LATIN POST.\r\nq http://www.eurotrends.it/en6.asp

Reply to this comment

physicsphysics
2011-10-11 08:21:33

I think this problem is a little bit tricky. To obtain the equation form of integral, the system should be changed reversibly. If this system is irreversible, the entropy change of this system is - $Delta q/T + \Delta q/T =$ zero.

physicsphysics
2011-10-11 08:24:13

Sorry. I confused two cases. Just reversible case is zero. irreversible case is S>=0.

Reply to this comment

mrTrig
2010-11-05 13:50:32

yosun, you can reduce the logarithms much faster by simply knowing that addition of two results in multiplication of arguments. 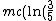$mc(\ln{(\frac{3}{5})} +\ln{3}) = mc\ln{(\frac{9}{5})}$

Reply to this comment

asdfman
2009-11-05 00:11:38

Reply to this comment

dstahlke
2009-10-09 10:53:51

But isn't it true that 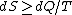$dS \ge dQ/T$ with equality only in the case where the process is reversible? This process doesn't seem reversible to me.

kroner
2009-10-11 20:16:00

In the context of the whole system it's not a reversible process. From that perspective dQ = 0 so you are correct that dS > dQ/T = 0. Clearly the change in entropy is positive.

But considering the two objects separately, they're each undergoing a reversible process (being uniformly heated or cooled). The change in entropy for each can be found by setting dS = dQ/T where dQ is the heat flowing into that object. Then you sum the changes contributed by each object.

Reply to this comment

vinograd19
2007-02-02 10:27:17

I think there is a mistake in solution. One integral is positive, the other is negative. But in solution they are both positive.

hungrychemist
2007-10-07 21:34:32

Solution is correct. The sign of integral is determined solely from the limits of integration. Notice ln(3/5) itself is a minus number as expected.

Reply to this comment

Post A Comment!

You are replying to:

Bare Basic LaTeX Rosetta Stone
LaTeX syntax supported through dollar sign wrappers $, ex., $\alpha^2_0$ produces $\alpha^2_0$ .
type this...	to get...
$\int_0^\infty$	$\int_0^\infty$
$\partial$	$\partial$
$\Rightarrow$	$\Rightarrow$
$\ddot{x},\dot{x}$	$\ddot{x},\dot{x}$
$\sqrt{z}$	$\sqrt{z}$
$\langle my \rangle$	$\langle my \rangle$
$\left( abacadabra \right)_{me}$	$\left( abacadabra \right)_{me}$
$\vec{E}$	$\vec{E}$
$\frac{a}{b}$	$\frac{a}{b}$

The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it...

All-Solutions List | Latest Comments | Unanswered Questions (Cries for Help!) |::| About

This site is not affiliated with ETS, nor is it officially endorsed (yet) by ETS. This site is the work of an individual named Yosun Chang. The solutions, sans user comments and ETS questions, are © 2005 by Yosun Chang except where noted. All rights reserved. / The comments appending particular solutions are due to the respective users. / Educational Testing Service, ETS, the ETS logo, Graduate Record Examinations, and GRE are registered trademarks of Educational Testing Service. The examination questions are © 1997 and © 2001 by ETS.

Bare Basic LaTeX Rosetta Stone