|
GR9277 #40
|
|
|
Problem
|
|
\prob{40}
A rigid cylinder rolls at constant speed without slipping on top of a horizontal plane surface. The acceleration of a point on the circumference of the cylinder at the moment when the paint touches the plane is
- directed forward
- directed backward
- directed up
- zero
|
Mechanics }Centripetal Force }Centripetal Force
There is no tangential acceleration (since otherwise it would slide and not roll---the frictional force balances the forward acceleration force). However, there is a centripetal acceleration that pulls the particles back in a circle, as in choice (C). This acceleration propels the tangential velocity to continue spinning in a circle.
|
|
|
Alternate Solutions |
alemsalem
2010-09-25 07:07:48 | for a cylinder rolling on horizontal surface without slipping, the center of mass moves with a constant speed, so the acceleration would be the same for a frame moving with the center of mass.
in the center of mass the cylinder is just rotating, which means the acceleration is toward the center,that is directed up
(C) |  |
|
|
Comments |
deneb
2018-10-13 21:57:38 | Damnit I thought this was rolling down an incline. So I added a downward acceleration due to gravity to the inward pointing centripetal acceleration and picked A... |  | epuma
2013-10-08 23:51:26 | Just remember, if a point is moving in a uniform circle, there is a net centripetal force keeping it there! Centripetal force and centripetal acceleration always point towards the center of the circle. Thus, the acceleration points upward.
Q: "But what about gravity or the ground?"
A: Even if gravity and the ground were to exert some force (they do!), the net force on the point MUST be towards the cylinder's center by the requirements of uniform circular motion. |  | Srivatsan
2012-11-05 02:20:35 | |  | alemsalem
2010-09-25 07:07:48 | for a cylinder rolling on horizontal surface without slipping, the center of mass moves with a constant speed, so the acceleration would be the same for a frame moving with the center of mass.
in the center of mass the cylinder is just rotating, which means the acceleration is toward the center,that is directed up
(C)
asa1985
2011-09-29 06:30:42 |
NiCe....
|
Allenji
2012-09-29 18:36:51 |
Nice solution, considering the superposition of acceleration is enough.
|
Srivatsan
2012-11-05 02:17:28 |
I have a question.
Since the point in contact with the plane is instantaneously at rest, how can it have a centripetal acceleration? The rotational motion that is equivalent to pure rolling is a pure rotation through the point of contact. The point of contact is the instantaneous centre of rotation. That being said, since the centre of rotation does not feel a centripetal force, the acceleration must be zero
|
|  | gabep
2009-09-26 04:59:11 | A point on a wheel/cylinder that rolls without slipping traces out a path called a cycloid. If you know what a cycloid looks like, you can draw it out and the direction of acceleration becomes obvious. http://en.wikipedia.org/wiki/Cycloid |  | chrisfizzix
2008-10-06 12:52:02 | Don't ever forget that you can always decompose this kind of motion into roation about the COM. and linear motion along the COM. Basically, the wheel is rotating about its COM and the whole thing is moving along with 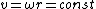 . So, the only acceleration of a point anywhere on the whole wheel is going to be its acceleration due to the rotational motion, which is just centripetal acceleration, always pointing inwards. Thus, for a point at the bottom of the wheel, up. . So, the only acceleration of a point anywhere on the whole wheel is going to be its acceleration due to the rotational motion, which is just centripetal acceleration, always pointing inwards. Thus, for a point at the bottom of the wheel, up. |  | Jeremy
2007-10-31 11:11:36 | 1. "paint" should be "point"
2. Answer choice (D) should be "directed down"
3. Answer choice (E) should be "zero" |  | explainthatagain
2006-12-12 11:59:08 | This is for everyone. I am studying alone and used google a bit. I found that OSU has a GRE prep course. How does this help? They put the couse lesson plan (lesson by lesson) online. The link is this. http://www.physics.ohio-state.edu/undergrad/ugs_gre.php?print=1 />
That lesson plan plus this website is what I am going to try. I will let you know when I take it in April 14th. Wish me luck.
eliasds@yahoo.com
2008-11-07 20:03:23 |
FYI, the questions on OSU's website are just taken from the 4 practice tests. I recommend taking the real practice tests before going back over OSU's "homework".
PS: Good luck to everyone taking the exam tomorrow!!!!!!!!
|
|  | Healeyx76
2006-10-31 13:54:36 | Its not asking the force on the whole cylinder, just on som imaginary point P on the outside. |  | senatez
2006-10-20 14:22:24 | I got confused as to the accelleration due to gravity. Do we not need to consider it?
evanb
2008-06-23 18:27:39 |
No, you need to consider the net motion of the point.
We can write down the position of a (well-chosen) point as
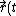 = \vec{r}_{center\ of\ cylinder}(t) + \vec{r}_{from\ center\ to\ point}(t))
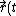 = (v t\hat{\i} R\hat{\j}) + R ( \sin{\omega t} \hat{\i} - cos{\omega t} \hat{\j} ))
Now, take the 2nd derivative. Notice that our point can be described by t = 0.
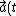 = 0 + 0 + R*(-\omega^2 \sin{\omega t} \hat{\i} + \omega ^2 \cos{\omega t} \hat{\j}))
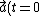 = 0 + 0 + 0 + R \omega ^2 \hat{\j})
So, the acceleration of the point that is at the bottom is directly up.
|
Poop Loops
2008-10-12 00:31:00 |
You can also remember that there is a normal force acting against gravity, giving you net zero acceleration.
If there WAS acceleration by gravity, the whole thing would be falling down by definition. But it just keeps rolling, so gravity isn't accelerating it.
|
|  |
|
|
|
|
The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it... |
|
|
|
