|
GR9277 #36
|
|
|
Problem
|
|
\prob{36}
A plane-polarized electromagnetic wave is incident normally on a flat, perfectly conducting surface. Upon reflection at the surface, which of the following is true?
- Both the electric vector and magnetic vector are reversed.
- Neither the electric vector nor the magnetic vector is reversed.
- The electric vector is reversed; the magnetic vector is not.
- The magnetic vector is reversed; the electric vector is not.
- The directions of the electric and magnetic vectors are interchanged.
|
Electromagnetism }Boundary Conditions }Boundary Conditions
The conductor perfectly reflects the incoming wave, and none is transmitted. The electric field is thus reversed. However, since E and B are perpendicular (related to each other by the Poyting Vector where the direction of propagation is given by the direction of 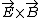 ), the magnitude of B is increased by 2, but its direction stays the same. ), the magnitude of B is increased by 2, but its direction stays the same.
Search on the GRE Physics Solutions homepage with keyword conductors for more on this.
|
|
|
Alternate Solutions |
Herminso
2009-09-13 17:12:50 | By BC's the parallel components of the electric field at the conducting boundary need to be continuous. Since the wave is incident normally on a flat, we know that the electric and magnetic field of the electromagnetic wave are parallel to the surface (Remember  ). So the BC for the electric field is: ). So the BC for the electric field is:
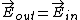
or
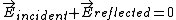
where 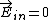 because the field inside to a conductor must be zero. Thus because the field inside to a conductor must be zero. Thus 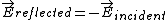 , the electric field is reversed. , the electric field is reversed.
Using now the relation  for the reflected wave, we can see the reflected magnetic field is not reversed. for the reflected wave, we can see the reflected magnetic field is not reversed.
|  |
|
|
Comments |
asdfasdfasdf
2016-08-09 16:43:11 | It\'s not too difficult for me to see that the electric field is reversed, but knowing that only rules out B and D, leaving 3 possibilities.\r\n\r\nCould A and E be ruled out together? Perhaps I\'m misunderstanding what E is saying, but it sounds like the same thing as A, and thus, they can\'t both be correct. If so, then C is left as the only option. |  | Herminso
2009-09-13 17:12:50 | By BC's the parallel components of the electric field at the conducting boundary need to be continuous. Since the wave is incident normally on a flat, we know that the electric and magnetic field of the electromagnetic wave are parallel to the surface (Remember  ). So the BC for the electric field is: ). So the BC for the electric field is:
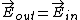
or
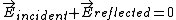
where 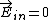 because the field inside to a conductor must be zero. Thus because the field inside to a conductor must be zero. Thus 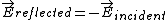 , the electric field is reversed. , the electric field is reversed.
Using now the relation  for the reflected wave, we can see the reflected magnetic field is not reversed. for the reflected wave, we can see the reflected magnetic field is not reversed.
Allenji
2012-09-29 18:30:48 |
concise and intuitive
|
Jovensky
2013-03-24 21:25:25 |
Excellent solution
|
gear3
2013-10-09 06:07:49 |
very good!
|
|  | flux
2008-11-07 18:16:24 | Because the surface is a conductor, the E field must go to zero. This is similar to a rope with a fixed end. The E field will thus flip over and head the other direction. This leaves us with choices A and C. Using the right-hand-rule brings us to the conclusion that the B field holds its direction. Choice C it is!
Almno10
2010-11-12 14:40:25 |
Likewise, the B is not necessarily zero at the surface, analogous to a rope that is ties but not fixed to a pole, so it does not change phase.
|
|  | Lukav
2008-10-03 12:42:32 | Kinda Silly, but you can just use the right hand rule to do this quickly.
Poop Loops
2008-10-05 15:38:38 |
Yes, but how do you know whether it's the E or B field that reversed?
|
|  | Furious
2007-10-29 15:41:36 | This probably is stupid, but why is it that the transverse portion of the E field must be reversed, is this the same reason why two problems prior to this the transverse E field had to be zero? I'm not quite sure which physical concept is responsible for this.
nick1234
2007-10-29 17:41:14 |
There can't be an electric field along the surface of a conductor, otherwise the free electrons would move along the surface until the E-field was canceled. So, any E-field that exists must be perpendicular to the surface. (Griffiths p.98)
|
Poop Loops
2008-10-05 15:37:29 |
In short, yes, exactly that reason. E|| has to go to zero at the edges, whereas B|| can stay the same, so it makes sense that it would be E to flip directions.
|
his dudeness
2010-09-04 13:02:20 |
Basically, the total transverse E-field must be zero, like Furious said (see two problems ago). How do we get that to happen? Well, given that the incoming wave has some transverse E-field E_t, the reflected wave must therefore have transverse E-field -E_t. That way, E_t + (-E_t) = 0 and the boundary condition is met.
|
|  |
|
|
|
|
The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it... |
|
|
|
