GREPhysics.NET: GR8677 #78

GR8677 #78

Problem

GREPhysics.NET Official Solution

Mechanics $\Rightarrow$ }Conservation of energy

There isn't much to say about this problem other than the fact that it's a conceptual conservation of energy problem. Particle 1 moves at velocity $v$ towards particle 2, initially at rest. EM-potential energy increases as they get closer and closer together---but, energy should still be conserved since no energy is radiated. The potential energy increase comes from the initial kinetic energy of particle 1. Take choice (C). (The other choices are all either too weird or just plain bogus.)

Alternate Solutions

Saint_Oliver
2013-09-13 20:08:00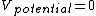

$V_{potential}=0$
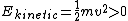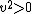
$E_{kinetic}=\frac{1}{2}mv^2\gt 0$ since $v^2\gt 0$

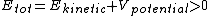
$E_{tot}=E_{kinetic}+V_{potential}\gt 0$

Therefore the total energy is greater than 0, which leaves only choice (C).

Reply to this comment

Comments

Saint_Oliver
2013-09-13 20:08:00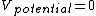

$V_{potential}=0$
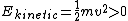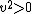
$E_{kinetic}=\frac{1}{2}mv^2\gt 0$ since $v^2\gt 0$

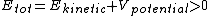
$E_{tot}=E_{kinetic}+V_{potential}\gt 0$

Therefore the total energy is greater than 0, which leaves only choice (C).

Reply to this comment

thebigshow500
2008-10-14 01:41:19

So what will happen if radiation involves? I can't get a concise answer from this case. Anyone care to explain?

Poop Loops
2008-11-05 21:21:34

Makes things a lot harder. Charged particles radiate when they accelerate or decelerate. Things with mass accelerate or decelerate under a force. So they'd be pushing each other away and radiating, etc. So it's weird.

Reply to this comment

grae313
2007-10-07 18:34:19

Yosun, you assume the particle with a velocity is moving towards the stationary particle, but what if it was moving away from the particle? The answer must be valid for both cases (moving towards the particle, or away), and in the case where it moves away, potential energy will always be zero and the total energy will just be given by the positive kinetic energy. Thus, C is clearly the answer.

511mev
2009-11-05 21:57:33

I know this is an old comment, but i'd still like to clarify: the potential energy is only zero at time zero, but since the coulomb potential is conservative, then T + V = a constant (of unspecified sign, since we don't know the signs of the charges).

llama
2013-10-16 18:19:15

T+V is a positive constant, since initially V=0 (as stated in the question) and T>0 (since .5*mv^2>0)

Reply to this comment

dbiggerstaff
2005-11-10 00:13:03

There\'s a simpler way to look at it: the problem says that each particle initially has zero potential energy, but that one particle has a velocity relative to the other, which implies that it will have some kinetic energy in this reference frame. This means the total initial energy is T+U = (something)+0 = (something positive.) Since there\'s no radiation, energy will be conserved, and the total energy will remain (something positive.) Thus, the correct answer is (c).

nitin
2006-11-16 13:36:49

Thumbs up mate!

abc111
2009-10-02 02:54:55

can anyone explain... the sign cannot be determined unless intial velocity is known.....i am thinking...T is only thing that contains velocity here...but it will absorb minus sign because 1/2 m v^2 has a square term...

kaic
2013-10-09 13:37:53

It's highly unlikely that the particles would have negative energy, don't you think? While negative energy is not forbidden, and has been demonstrated to be possible, it's unlikely to show up on the GRE unless it is explicitly included as part of a question. As you said, the sign of the initial velocity does not matter because it is squared in the kinetic energy, so T is positive, and U is 0, so total energy E = T + V is positive.

Reply to this comment

Post A Comment!

You are replying to:

$V_{potential}=0$ $E_{kinetic}=\frac{1}{2}mv^2\gt 0$ since $v^2\gt 0$ $E_{tot}=E_{kinetic}+V_{potential}\gt 0$ Therefore the total energy is greater than 0, which leaves only choice (C).

Bare Basic LaTeX Rosetta Stone
LaTeX syntax supported through dollar sign wrappers $, ex., $\alpha^2_0$ produces $\alpha^2_0$ .
type this...	to get...
$\int_0^\infty$	$\int_0^\infty$
$\partial$	$\partial$
$\Rightarrow$	$\Rightarrow$
$\ddot{x},\dot{x}$	$\ddot{x},\dot{x}$
$\sqrt{z}$	$\sqrt{z}$
$\langle my \rangle$	$\langle my \rangle$
$\left( abacadabra \right)_{me}$	$\left( abacadabra \right)_{me}$
$\vec{E}$	$\vec{E}$
$\frac{a}{b}$	$\frac{a}{b}$

The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it...

All-Solutions List | Latest Comments | Unanswered Questions (Cries for Help!) |::| About

This site is not affiliated with ETS, nor is it officially endorsed (yet) by ETS. This site is the work of an individual named Yosun Chang. The solutions, sans user comments and ETS questions, are © 2005 by Yosun Chang except where noted. All rights reserved. / The comments appending particular solutions are due to the respective users. / Educational Testing Service, ETS, the ETS logo, Graduate Record Examinations, and GRE are registered trademarks of Educational Testing Service. The examination questions are © 1997 and © 2001 by ETS.

Bare Basic LaTeX Rosetta Stone