|
GR0177 #38
|
|
|
Problem
|
|
|
This problem is still being typed. |
Electromagnetism }AC Circuit }AC Circuit
The current is maximized when one has resonance. Resonance occurs when the complex impedance is 0, or when 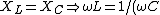) . Plug stuff in to get choice (D). . Plug stuff in to get choice (D).
|
|
|
Alternate Solutions |
| There are no Alternate Solutions for this problem. Be the first to post one! |
|
|
Comments |
giga17
2010-08-11 02:06:05 | This is one of those problems where dimensional analysis would help a lot. The only way to get a time scale out of the physical quantities from R, L and C is 1/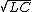 . .
acukierm
2013-09-23 19:41:23 |
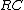 also has units of time. So pure dimensional analysis with no other information (e.g., that the inductor is important for finding the resonance frequency while the resistor is not) would not work here. also has units of time. So pure dimensional analysis with no other information (e.g., that the inductor is important for finding the resonance frequency while the resistor is not) would not work here.
|
|  | zmb_hep
2009-09-08 15:36:21 | One can also use the resonant frequency condition 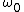 =1/ =1/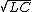 . .
We are given that 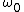 =10^3 , and that L=25 mH, plugging these values in and solving for C also gives the correct answer =10^3 , and that L=25 mH, plugging these values in and solving for C also gives the correct answer
dberger8
2016-01-15 22:14:52 |
We\\\\\\\'re given the driving frequency, not the natural frequency.
|
jfcdu
2018-07-15 14:40:57 |
@dberger8\\\\r\\\\n the idea, is that the driving frequency is set by the problem. If we want to maximize the amplitude of the current, we need to pick C such that the resonant frequency *is* the driving frequency. Thus we can set 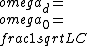 and solve for C. and solve for C.
|
jfcdu
2018-07-15 14:43:27 |
@dberger8 the idea is that the driving frequency is set by the problem. If we want to maximize the amplitude of the current, we need to pick C such that the resonant frequency *is* the driving frequency. Thus we can set 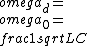 and solve for C. and solve for C.
|
|  |
|
| Post A Comment! |
|
|
Bare Basic LaTeX Rosetta Stone
|
LaTeX syntax supported through dollar sign wrappers $, ex., $\alpha^2_0$ produces  . .
|
| type this... |
to get... |
| $\int_0^\infty$ |
 |
| $\partial$ |
 |
| $\Rightarrow$ |
 |
| $\ddot{x},\dot{x}$ |
 |
| $\sqrt{z}$ |
 |
| $\langle my \rangle$ |
 |
| $\left( abacadabra \right)_{me}$ |
_{me}) |
| $\vec{E}$ |
 |
| $\frac{a}{b}$ |
 |
|
|
|
|
The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it... |
|
|
|
